Mathematics Dictionary Dr. K. G. Shih

---

Locus of geometrical diagrams

Subjects

Symbol Defintion Example : Sqr(x) = Square root of x

GE 10 00 | - Outlines GE 10 01 | - Diagrams GE 10 02 | - Locus of in-center GE 10 03 | - Locus of centroid GE 10 04 | - Locus of circum-center GE 10 05 | - Locus of ex-center GE 10 06 | - Locus of ortho-center GE 10 07 | - GE 10 08 | - GE 10 09 | - GE 10 10 | -

Answers

GE 10 01. Diagrams Diagram program Geometry | Section 10 : Locus Procedures 1. Click above program Screen 1 : Select run at current location (No download) Screen 2 : Select yes to run 2. Click Menu command 3. Click section 10 in upper box 4. Click a program in lower box 5. Read the question 6. Click Re-plot to see demo Go to Begin GE 10 02. Locus of in-center Question Two fixed points A and B One moving point C keeps angle ACB as constant What is the locus of the in-center of triangle ABC Diagram Text Angle AIB = pi - A/2 - B/2 = pi + C/2 Hence angle AIB = constant Since angle AIB is constant A and B are fixed and then locus of in-center I is an arc Demo Diagram Geometry | Section 10 : Locus Text of in-center In-center Theory Proof in Q04 Go to Begin GE 10 03. 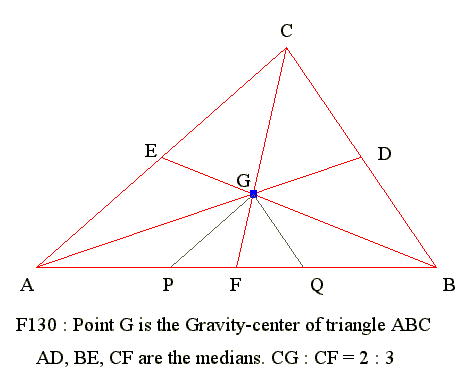Locus of gravity center Question Two fixed points A and B One moving point C keeps angle ACB as constant What is the locus of the centroid of triangle ABC Diagram Text Draw GP parallel to AC and GQ parallel to BC Hence angle PGQ = angle ACB = constant Since angle PGQ is constant P and Q are fixed and then locus of centoid G is an arc Demo program Geometry | Section 10 : Locus Text of in-center gravity-center Theory Proof in Q04 Go to Begin GE 10 04. Locus of circum-center Question Two fixed points A and B One moving point C keeps angle ACB as constant What is the locus of the circum-center of triangle ABC Diagram Text Since point C is on the same circle Hence point E will keep at same position Diagram program Geometry | Section 10 : Locus Text of in-center circum-center Theory Proof in Q04 Go to Begin GE 10 05. Locus of ex-center Question Two fixed points A and B One moving point C keeps angle ACB as constant What is the locus of the ex-center of triangle ABC Diagram Text Angle AEB = pi - A/2 - B - (pi - B)/2 = pi - (A + B)/2 = pi/2 + C/2 Hence angle AEB = constant Locus of ex-center is an arc Diagram program Geometry | Section 10 : Locus Text of in-center Ex-center Theory Proof in Q04 Go to Begin GE 10 06. Locus of ortho-center Question Two fixed points A and B One moving point C keeps angle ACB as constant What is the locus of the ortho-center of triangle ABC Diagram Text Angle ADC = right angle Angle BEC = right angle Hence AOB = pi - angle ACB and angle ACB is constant Since angle ACB is constant. Hence angle AOB is constant A and B are fixed and then locus of ortho-center O is an arc Demo program Geometry | Section 10 : Locus Go to Begin GE 10 07. Answer Question Diagram program Geometry | Section 10 : Locus Text Go to Begin GE 10 08. Answer Go to Begin GE 10 09. Answer Go to Begin GE 10 10. Answer Go to Begin GE 10 00. Outlines GE 10 01 : The use of diagrams in Geom.exe Definition A and B are fixed points. Point C moves with constant angle ACB, then locus of C is arc Locus of five centers of triangle If points A and B are fixed and point C moves with constant angle what are the locus of the centers ? Go to Begin

---

Show Room of MD2002

Contact Dr. Shih

Math Examples Room

---

Copyright © Dr. K. G. Shih, Nova Scotia, Canada.

---